Blog

Introduction

Risk is an unavoidable part of life and is particularly embedded when determining the cost of capital. No investment or valuation is guaranteed, as any prudent advisor or planner will tell you, but quality analysts and investors try to quantify and forecast risk.

The core output of the valuation process’ cost of capital is essentially a “cost of risk,” or the total premium expected for investing in an asset or a stake in a company. Proxies for risk drive the cost of risk output – namely, the firm’s particular beta and the market-wide, systematic risk. We will start with the latter.

Systematic Risk

Capital market theory divides risk into two baskets: systematic and unsystematic (also called idiosyncratic risk). Systematic risk is a market-wide risk that’s unplanned, unforeseen, and affects all participants (more or less) equally. Systematic events include natural disasters, economic events like interest rate hikes or large-scale crises, as in 2008, and any other widespread event that affects broad market returns. Unsystematic risk impacts smaller slices of the market, like individual firms or specific industries.

In most cases, systematic risks are not diversifiable, as they affect all players in the market equally, whereas unsystematic risks do not. For example, the early days of the pandemic affected the global markets’ return, including airline stocks, as a systematic risk. In contrast, United Airlines’ 7% drop in 2017 after a passenger was dragged from a flight was unsystematic. An investor holding an index fund and United stock would have seen the latter portion tank in 2017 while the market index remained unaffected, but both halves of the portfolio would suffer in March 2020.

It’s important to note that although the systematic risk is not diversifiable, it is insurable and these two characteristics should not be conflated. Although an investor can hedge against particular market-wide possibilities, he cannot explicitly predict them or buy a basket of diversified assets unaffected by the events. An investor with a position in SPY puts or VIX calls, for example, profits from the insurance in the event of a market crash. But, since those positions are still affected by the systematic risk, albeit to his benefit, they haven’t diversified away from the risk itself.

In a cost of capital calculation, we’re finding the premium between accepting undiversified market risk (the investment or company we’re valuing) and investing in alternative assets such as a diversified market portfolio or a risk-free asset. While that premium between the market-wide risk and the risk-free rate is an equity risk premium, we must find to what degree the actual company is affected by typical market volatility. That input to the model is the company’s beta.

Beta

The beta is the degree to which the market informs a stock’s volatility when compared to the “market as a whole”. The market beta is 1 by default, so any company with a beta above 1 generates greater returns than the market when the market does well, and lower returns when the market does not do well. If the difference between the stock or investment’s return is less than the market, the beta is less than 1. As an example, large supermarket chains have a beta less than one (less risky and hence less return on investment when compared to the market). A tech stock has higher volatility and higher returns than the market (when the market does well).

Analysts use beta to measure the effect of systematic market risks on the company so investors have the possibility to hedge their portfolio against the risk since they cannot otherwise diversify the risk away. Using a simple example, a volatile firm in the tech sector’s beta is 1.5. If an investor were bullish on the company but wanted to lower overall portfolio risk, they would be short $500 in the overall market for every $1,000 invested in the stock. Shorting in relation to a long investment would dampen the effects of risk on the portfolio, although it would not negate it.

It is important to note that beta alone is insufficient to fully assess the firm’s risk and volatility, as it only measures correlation with the market. Some stocks and industries have a low correlation with the market and a low beta but are highly volatile due to unsystematic factors. For example, many biotech stocks have betas around one (1). Still, they are highly volatile as their pricing is more informed by FDA decisions and other industry-specific risks than the overall market.

Depending on whether the firm is publicly traded or private, drives different beta estimate techniques. Public betas are relatively straightforward to estimate because, although the beta is a forward-looking measure, beta could be approximated by measuring the stock’s past performance against historical market performance. Public firms also have disclosed capital structures, so we have a holistic measure of the effects of debt and equity on its volatility.

In valuation and cost of capital for private firms, betas are calculated by comparing public company beta averages in the same industry. But the technique is more complex than taking the mean of a handful of similar companies. Assuming we know the private company’s capital structure, we compare its debt-to-equity ratio against a set of guidelines and standards for that industry. If it differs substantially, we must unlever the public betas as if they had no debt, then relever per the private firm’s capital structure.

​​Unlevering and Relevering Beta

If determining the cost of capital for a private company with capital structuring or perspectives on leveraging that differ from publicly traded benchmark betas, the beta must be unlevered and realigned with a more accurate debt/equity structure for appropriate use. An unlevered beta accomplishes the same goal as a standard beta but negates the impact of debt on a company’s capital structure. This negation lets analysts see the volatility, or risk, of the company’s equity and assets.

There are two standard formulas used to unlever and relever betas when calculating a cost of capital: the Hamada and Harris-Pringle formulas.

Hamada Formula

In this calculation:

• βU = beta unlevered
• βL = Beta levered
• t = Tax rate for the company
• Wd = Percentage of debt in the capital structure
• We = Percentage of equity in the capital structure

Once an analysts pulls a new, unlevered beta from the Hamada formula, it’s relevered to realign with the appropriate capital structure:

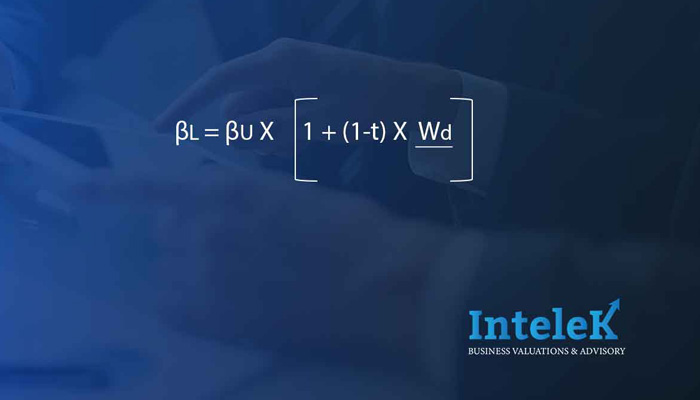
The Hamada formulas operate under an analyst’s bias that:

1. there is little to no risk of defaulting on debt
2. both interest and principal payments will follow their respective schedule without a hitch
3. the debt also maintains the same net balance from the date an analyst calculates the unlevered beta

Furthermore, it (correctly or not) assumes that debt and the tax shield’s discount rate is equal to the cost of debt itself. In other words, the tax shield carries the same risk as debt.

In short, the Hamada formula is excellent for MBA students and budding analysts, but its blue-sky perspective doesn’t hold well in the real world.

Harris-Pringle Formula

To counter inaccurate, generalized assumptions required in the Hamada formula, valuation experts use the Harris-Pringle formula as an alternative.

The variables remain unchanged from the Hamada method, but astute readers recognize one addition:

Because debt is assigned a beta of its own using the Harris-Pringle formula, it more accurately accounts for the riskiness inherent in carrying debt and leverage. The debt beta accounts for the likelihood, however slim, that either interest or principal payments go unpaid or are paid late. This variability affects the tax benefits of interest payments, so it is more applicable to corporate finance and tax management practices.

The Harris-Pringle formula is also more practical when approaching debt load, as it assumes the proportion of debt to equity remains fixed rather than the net debt balance itself. This assumption is more aligned with typical capital structuring goals that seek to maintain proportionality, as keeping a steady balance is impractical in the real world.

Beta Management

When used as a valuation input, we must assume that the capital structuring for public benchmark firms and the private subject company are valued at their market value when calculated. Therefore, analysts must carefully manage and oversee the impacts of both cost of equity and debt in the cost of capital inputs and how unlevering and relevering beta affect each. Otherwise, the proportion may be affected and skew the end valuation results.

Measuring beta is an incomplete method of assessing volatility, particularly when valuing private companies. Since it is based on historical outcomes but applied predictively, there needs to be more specificity to mitigate or manage future events. Analysts use additional risk premia factors in a cost of capital calculation to manage both.